---
title: "Lightsaber Capability Analysis: Is Our Process In Control?"
description: "Lightsaber Capability Analysis: Is Our Process In Control?"
image: https://blog.minitab.com/hubfs/B2BML%20-%20NEW%20FILE%20MANAGER%20STRUCTURE/Blog%20Images/Imported_Blog_Media/lightsabers-3.gif
---
English
- [English](https://blog.minitab.com/en/?hsLang=en)
- [Français](https://blog.minitab.com/fr/?hsLang=en)
- [Deutsch](https://blog.minitab.com/de/?hsLang=en)
- [Português](https://blog.minitab.com/pt/?hsLang=en)
- [Español](https://blog.minitab.com/es/?hsLang=en)
- [日本語](https://blog.minitab.com/ja/?hsLang=en)
- [한국어](https://blog.minitab.com/ko/?hsLang=en)
- [中文（简体）](https://blog.minitab.com/zh/?hsLang=en)
- [ Partners](https://www.minitab.com/en-us/company/partners/)
- [ Contact](https://www.minitab.com/en-us/company/contact-us/)
- [ Talk to Minitab](https://www.minitab.com/en-us/talk-to-minitab/)
- [ My Account](https://licensing.minitab.com/account/manage)
[](https://www.minitab.com/en-us/)
- Products
Back
[Products](https://www.minitab.com/en-us/products/)
[All Products](https://www.minitab.com/en-us/products/)
- [Minitab Solution Center](https://www.minitab.com/en-us/products/minitab-solution-center/)
- [Minitab Statistical Software](https://www.minitab.com/en-us/products/minitab/)
- [Minitab Connect](https://www.minitab.com/en-us/products/connect/)
- [Minitab Model Ops](https://www.minitab.com/en-us/products/model-ops/)
- [Minitab Education Hub](https://www.minitab.com/en-us/products/education-hub/)
- [Minitab Engage](https://www.minitab.com/en-us/products/engage/)
- [Minitab Workspace](https://www.minitab.com/en-us/products/workspace/)
- [Real-Time SPC](https://www.minitab.com/en-us/products/real-time-spc/)
- [Prolink Data Collection & SPC](https://www.minitab.com/en-us/products/prolink)
- [Simul8 Discrete Event Simulation](https://info.minitab.com/en/new-to-simul8?hsLang=en)
- [SPM](https://www.minitab.com/en-us/products/spm/)
- Solutions
Back
[Solutions](https://www.minitab.com/en-us/solutions/)
[All Solutions](https://www.minitab.com/en-us/solutions/)
- [Analytics](https://www.minitab.com/en-us/solutions/analytics/)
- [Statistics & Predictive Analytics](https://www.minitab.com/en-us/solutions/analytics/statistical-analysis-predictive-analytics/)
- [Data Science & Machine Learning](https://www.minitab.com/en-us/solutions/analytics/data-science-machine-learning/)
- [Business Analytics & Intelligence](https://www.minitab.com/en-us/solutions/analytics/business-analytics-intelligence/)
- [Statistical Process Control](https://www.minitab.com/en-us/solutions/analytics/statistical-process-control/)
- [Quality Analytics](https://www.minitab.com/en-us/solutions/analytics/quality-analytics/)
- [Live Analytics](https://www.minitab.com/en-us/solutions/analytics/live-analytics/)
- [Reliability & Life Data Analysis](https://www.minitab.com/en-us/solutions/analytics/reliability/)
- [Discrete Event Simulation](https://info.minitab.com/en/new-to-simul8?hsLang=en)
- [Process Mining](https://info.minitab.com/en/new-to-simul8?hsLang=en)
- [Key Capabilities](https://www.minitab.com/en-us/solutions/key-capabilities/)
- [Continuous Improvement](https://www.minitab.com/en-us/solutions/key-capabilities/continuous-improvement/)
- [Data Integration & Data Prep](https://www.minitab.com/en-us/solutions/key-capabilities/data-integration-data-prep/)
- [Diagramming & Mind Mapping](https://www.minitab.com/en-us/solutions/key-capabilities/diagramming-mind-mapping/)
- [Digital Twins](https://info.minitab.com/en/new-to-simul8?hsLang=en)
- [Model Deployment & ML Ops](https://www.minitab.com/en-us/solutions/key-capabilities/model-deployment-ml-ops/)
- [Innovation & Project Management](https://www.minitab.com/en-us/solutions/key-capabilities/innovation-project-management/)
- [Process Excellence: Detect, Correct & Prevent](https://www.minitab.com/en-us/solutions/key-capabilities/process-excellence/)
- [Featured Industries](https://www.minitab.com/en-us/solutions/industry/)
- [Academic](https://www.minitab.com/en-us/solutions/industry/academic/)
- [Energy & Natural Resources](https://www.minitab.com/en-us/solutions/industry/energy-resources/)
- [Government & Public Sector](https://www.minitab.com/en-us/solutions/industry/government/)
- [Healthcare](https://www.minitab.com/en-us/solutions/industry/healthcare/)
- [Insurance](https://www.minitab.com/en-us/solutions/industry/insurance/)
- [Manufacturing & Industrial](https://www.minitab.com/en-us/solutions/industry/manufacturing-industrial/)
- [Services](https://www.minitab.com/en-us/solutions/industry/service-industries/)
- [Software & Technology](https://www.minitab.com/en-us/solutions/industry/software-technology/)
- [Construction](https://www.minitab.com/en-us/solutions/industry/construction/)
- [Featured Roles](https://www.minitab.com/en-us/solutions/role/)
- [Engineering](https://www.minitab.com/en-us/solutions/role/engineering/)
- [Business Analysis](https://www.minitab.com/en-us/solutions/role/business-analysts/)
- [Information Technology](https://www.minitab.com/en-us/solutions/role/information-technology/)
- [Supply Chain](https://www.minitab.com/en-us/solutions/role/supply-chain/)
- [Customer Service & Contact Center](https://www.minitab.com/en-us/solutions/role/customer-service-contact-center/)
- [Human Resources](https://www.minitab.com/en-us/solutions/role/human-resources/)
- [Marketing](https://www.minitab.com/en-us/solutions/role/marketing/)
- [Research and Development](https://www.minitab.com/en-us/solutions/role/research-and-development/)
- Resources & Services
Back
[Resources & Services](https://www.minitab.com/en-us/resources-services/)
[All Resources & Services](https://www.minitab.com/en-us/resources-services/)
- [Resources](https://www.minitab.com/en-us/resources-services/resources/)
- [Case Studies](https://www.minitab.com/en-us/resources-services/resources/case-studies/)
- [eBooks & White Papers](https://www.minitab.com/en-us/resources-services/resources/ebooks-and-white-papers/)
- [Blog](https://blog.minitab.com/blog/?hsLang=en)
- [Data Sets](https://www.minitab.com/en-us/resources-services/resources/data-set/)
- [Webinars & Events](https://www.minitab.com/en-us/resources-services/resources/events/)
- [Education Hub](https://www.minitab.com/en-us/resources-services/resources/education-hub/)
- [Services](https://www.minitab.com/en-us/resources-services/services/)
- [Training](https://www.minitab.com/en-us/resources-services/services/training/)
- [Deployment](https://www.minitab.com/en-us/resources-services/services/deployment/)
- [Consulting](https://www.minitab.com/en-us/resources-services/services/statistical-consulting/)
- [Self-Paced Learning](https://www.minitab.com/en-us/resources-services/services/self-paced-learning/)
- [Continuing Education](https://www.minitab.com/en-us/resources-services/services/continuing-education/)
- Support
Back
[Support](https://www.minitab.com/en-us/support/)
[Technical Support](https://www.minitab.com/en-us/support/)
- [Subscriptions & Activation](https://www.minitab.com/en-us/support/subscriptions-and-activation/)
- [Minitab Quick Start](https://www.minitab.com/en-us/support/quick-start/)
- [Training](https://www.minitab.com/en-us/resources-services/services/training/)
- [Installation Support](https://www.minitab.com/en-us/support/installation/)
- [Support Videos](https://www.minitab.com/en-us/support/videos/)
- [Support Documentation](https://www.minitab.com/en-us/support/documents/)
- [Software Updates](https://www.minitab.com/en-us/support/software-updates/)
- [Product Downloads](https://www.minitab.com/en-us/support/downloads/)
- [Support Policy](https://www.minitab.com/en-us/support/policy/)
- Company
Back
[Company](https://www.minitab.com/en-us/company/)
[Company](https://www.minitab.com/en-us/company/)
- [About Us](https://www.minitab.com/en-us/company/our-company/)
- [Leadership Team](https://www.minitab.com/en-us/company/leadership-team/)
- [Partners](https://www.minitab.com/en-us/company/partners/)
- [Careers](https://www.minitab.com/en-us/company/careers/)
- [Contact](https://www.minitab.com/en-us/company/contact-us/)
- [News](https://www.minitab.com/en-us/company/press-releases/)
[Try/Buy](https://www.minitab.com/en-us/try-buy/)
- [Statistics](https://blog.minitab.com/en/blog/tag/statistics)
- [Data Analysis](https://blog.minitab.com/en/blog/tag/data-analysis)
-
- [Quality Improvement](https://blog.minitab.com/en/blog/tag/quality-improvement)
- [Subscribe](https://info.minitab.com/blog/subscribe?hsLang=en)
## Notice
You are now leaving minitab.com.
Click Continue to proceed to:
Continue
Go Back
# Lightsaber Capability Analysis: Is Our Process In Control?
[Eric Heckman](https://blog.minitab.com/en/blog/author/eric-heckman) | 2/22/2013
Topics: [Capability Analysis](https://blog.minitab.com/en/blog/tag/capability-analysis), [Quality Improvement](https://blog.minitab.com/en/blog/tag/quality-improvement)
- [Share](https://www.linkedin.com/shareArticle?mini=true&url=https://blog.minitab.com/en/blog/starting-out-with-statistical-software/lightsaber-capability-analysis-is-our-process-in-control)
- [Share](https://www.facebook.com/sharer.php?u=https://blog.minitab.com/en/blog/starting-out-with-statistical-software/lightsaber-capability-analysis-is-our-process-in-control)
In my last post, we talked about using [statistical tools to identify the right distribution](https://blog.minitab.com/blog/starting-out-with-statistical-software/lightsaber-capability-analysis-picking-the-right-distribution?hsLang=en) of our lightsaber manufacturing data. Now that we have our data in Minitab along with a specific distribution picked out, we can find out if we are dealing with an in-control process. If the process is not in control, the capability estimates will be incorrect. Thus, an extremely important (and often overlooked) aspect of Capability Analysis is to make sure our process in first in control. We can do this with a tool Minitab [Statistical Software](http://www.minitab.com/products/minitab) offers called the Capability Sixpack.TM
First, let’s go to **Stat > Quality Tools > Capability Sixpack > Normal**. We’ve gotten our spec limits from the Jedi Temple, and know that they require the lightsabers to be between 2.75 to 3.25 feet in length. We also know that we have a target length of 3 feet. Knowing this information, we can fill out the dialog as follows:
We also want to tell Minitab that our measurements have a target we are trying to hit. Click the ‘Options’ button and enter a target of 3. Click OK in each menu, and our Capability Sixpack will be displayed.
Let’s see whether our process is in-control. Let’s first take a look at the Xbar Chart. We want to see the points randomly distributed between the control limits. This implies a stable process. The R chart works the same way. Again, we are looking to see the points randomly distributed between the control limits.
Looking at both of our charts in the Capability Sixpack, they seem to fit the conditions of a stable process. This is what we’re looking for. If our process isn’t stable, our capability analysis results can’t be trusted.
We should also compare points on the R chart with those on the Xbar chart to see if the points follow each other. If the process is stable, they shouldn't. These points do not follow each other, which again implies a stable process.
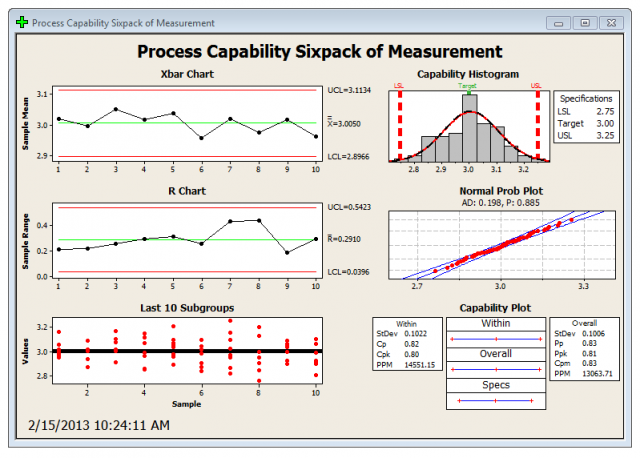
We also want to look at the Last 10 Subgroups Graph. Here, we're looking for a random, horizontal scatter, with no apparent shifts or drifts in the data. Shifting and drifting indicates a process that is out of control. Looking at our graph, the scatter appears to be randomly spread out, with no trends sticking out anywhere. All of our graphs seem to indicate that we have an in-control, stable graph. This is exactly what we are looking for.
If you want the interpretation of the process capability statistics to be valid, your data should [approximately follow a normal distribution](https://blog.minitab.com/blog/starting-out-with-statistical-software/lightsaber-capability-analysis-picking-the-right-distribution?hsLang=en), as I discussed in my last blog post. On the capability histogram, the data approximately follow a normal curve. In addition, the points on the normal probability plot approximately follow a straight line and fall within the 95% confidence interval. These patterns indicate that the data are normally distributed, and confirm what we found in the last post, which was that our data are indeed normal.
Taking into account that our data appear to be normal, and follow an in control, stable process, we are clear to go ahead and conduct a Capability Analysis whose results we can fully trust.
Next post, we'll perform the analysis and look in detail about what our capability analysis results tell us about lightsaber production, and whether we are meeting the standards that have been set for us by the Jedi Temple.
## You Might Also Like
[Do You Really Need That New Machine? How Minitab Can Help You Find Out](https://blog.minitab.com/en/blog/do-you-really-need-that-new-machine-how-minitab-can-help-you-find-out?hsLang=en)
[Quality Improvement](https://blog.minitab.com/en/blog/tag/quality-improvement) | 4 Minute Read
[How to Kickstart a Quality Program the Right Way](https://blog.minitab.com/en/blog/how-to-kickstart-a-quality-program-the-right-way?hsLang=en)
[Prolink](https://blog.minitab.com/en/blog/tag/prolink) | 2 Minute Read
[Moving From Lab Coat to Launch Pad in R&D](https://blog.minitab.com/en/blog/moving-from-the-lab-coat-to-the-launch-pad-in-rd?hsLang=en)
[Research And Development](https://blog.minitab.com/en/blog/tag/research-and-development) | 7 Minute Read
---
- [Careers](https://www.minitab.com/en-us/company/careers/)
- [Trust Center](https://www.minitab.com/en-us/trust-center/)
- [Suppliers](https://www.minitab.com/en-us/suppliers/)
- [Site Map](https://www.minitab.com/en-us/site-map/)
- [](https://www.linkedin.com/company/minitab/)
- [](https://www.facebook.com/Minitab/)
- [](https://www.youtube.com/user/MinitabInc)
- [](https://www.instagram.com/minitab/)
---
© 2025 Minitab, LLC. All Rights Reserved.
- [Terms of Use](https://www.minitab.com/en-us/legal/terms-of-use/)
- [Privacy Policy](https://www.minitab.com/en-us/legal/privacy-policy/)
- [Legal](https://www.minitab.com/en-us/legal/)
- Cookies Settings